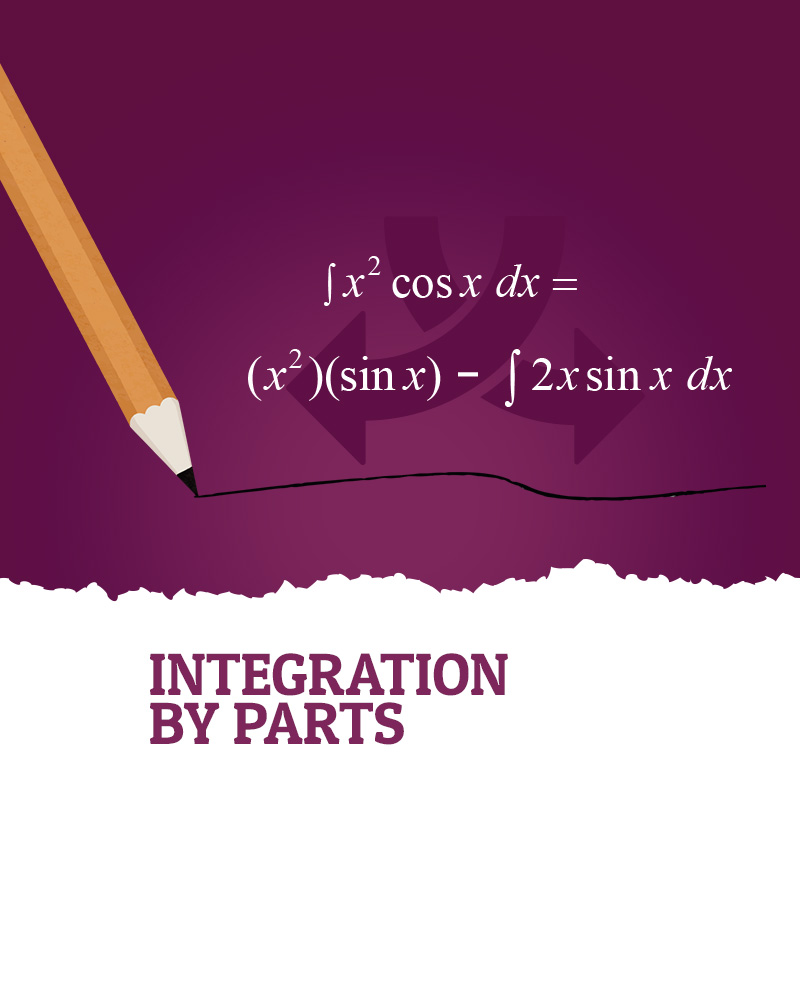
In this example, there is a product of x^2 and cosx dx in the integrand.
Does simple u-substitution method work for this example?
In order to use the simple u-substitution method, the relationship between two functions must be the original and its derivative each other except for constants.
Unfortunately, x^2 cannot be the derivative of cosx or cosx cannot be the derivative of x^2 either. Therefore, we cannot use the u-substitution method here.
Then Let’s try to use Integration by parts method.